How does this vary from the normal Super Bowl Squares?
The concept is the same, the difference is there are not four quarters in a college basketball game but instead two halves, therfore there will only be 2 winners, one at the end of the first half and one at the end of the second half. The best time to run this pool is for the Ncaa Tournament Championship game, but it can be ran for any game.

How does this vary from the normal 100 Square Grid?
Everything is the same but each square will now have two numbers in the left hand column, which gives each square two chances to match the team listed on the left side.

Step 1
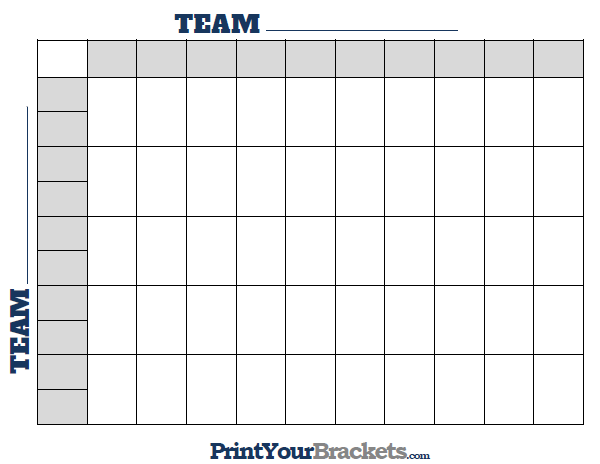
Print the square grid from above and place one of the teams on the top blank line and the other team on the side blank line. It doesn't matter which team goes where.

Step 2
Sell each square for a set dollar amount until all squares are full, a player may buy as many squares as they wish. Once the player pays for the squares they are to write their name in the squares of their choice. If you feel 50 squares is too few or too many check out our 25 square grid or 100 square grid.

Step 3
Once all the squares are full it is time to set up the drawing. This can be done in many ways, listed below are 2 examples. After determining the drawing method, draw the numbers one at a time placing the numbers from left to right starting with the first gray square box in the top row, continue across the top row until the numbers are gone. After that is complete you will redraw the numbers, this time placing the drawn numbers in the gray square boxes in the left column, starting at the top.
Example 1: Write the numbers 0-9 on pieces of paper and place them in a hat.
Example 2: Get a deck of cards A through 10, the ace represents a one, the 10 represents a 0, 2-9 are face value.

Step 4
Now is time for the fun!! Begin watching the game, at the end of each half match the last digit of each teams score with the grid.
Example: At the end of the first half if the team listed up top has 17 and the team listed on the left side has 14. Go to the top row of numbers and find the number 7(last digit of 17) then go to the left column of numbers and find the number 4(last digit of 14) find where these two numbers intersect on the grid and the name in that square wins the first half.